Ground
Works

Unfolding the Genome

Gupi Ranganathan*, Aiden Lab, Erez Lieberman Aiden*
*These authors contributed equally.



Abstract

From 2009-2011, we worked together at the Broad Institute of MIT and Harvard (Broad Institute, n.d.), building on a study (Lieberman-Aiden & Van Berkum et al., 2009) that made it possible to explore how the human genome, the DNA contained in every cell of the body, folds in 3D. At the outset of our collaboration, our approaches seemed so different as to be, perhaps, incommensurable. The scientists used tools like mathematics, computer science, and molecular biology, whereas the artistic toolkit was focused on the construction of physical objects, with a defined shape, area, and volume. Yet over time, we came to realize that all of these tools were addressing the same goal: making invisible concepts manifest as an experience intelligible to the senses. From the beginning of the project, we worked together creating drawings. Over time, our interactions evolved to become free-flowing conversations while drawing, which became a way of seeing together. Our visual experimentations grew into a body of drawings, paintings, prints, mixed-media artworks, wood blocks, a dynamic video installation, and a suspended wire sculpture (Ranganathan, 2021), and helped advance the scientific community’s understanding of how the human genome folds.

Unfolding the Genome

Published:

November 2, 2021 (1467 views *)

License:


Unfolding the Genome © 2021 by Gupi Ranganathan, Aiden Lab, and Erez Lieberman Aiden is licensed under CC-BY-NC-ND 4.0

Project Metadata:

Project Site · Sites and Institutions

Background

When we began our work together in 2009, we were aware of other arts-science explorations of the genome such as Ecce Homology, a collaborative group of artists and scientists working at the intersection of comparative genomics and immersive experience, and the research done by choreographer Liz Lerman in collaboration with researchers at the NIH, Johns Hopkins University, Stanford University, Howard University, the Genetics and Public Policy Center, the Institute for Genomic Research, and the U.S. Department of Energy. The scope and achievements of these projects inspired us; however, we found our tools (drawing, dance, genomic architecture, sculpture) and our specific area of inquiry (the structure of the genome as it folds and unfolds) to be entirely separate from theirs.

Gupi: From 2009-2011, as the second artist-in-residence at the Broad Institute of MIT and Harvard, I worked closely with Erez Lieberman Aiden, collaborating with him and his team on the genome folding project. Erez had just published a paper (Lieberman-Aiden & Van Berkum et al., 2009) in which he and colleagues developed a new method for deciphering the 3-D architecture of the human genome. In that setting, Erez and his collaborators proposed a model for how the genome folds that is based on so-called space-filling curves, such as the Hilbert (Fig. 1) and Peano curves (Fig. 2).

Could I as an artist advance such a research project? When Erez used the century-old mathematical theory of space-filling curves as a way to explain how the two-meter-long human genome folds itself inside a tiny space without tangling (Clegg, 2012), I did not know how I would be able to contribute.

Figure 1. Using the fractal globule model and the Hilbert Curve to explain 3-dimensional genome architecture. The fractal globule at the left is formed from a single, long, linear contour that has folded back upon itself. Positions that are nearby along the 1D linear contour have a similar color; the clustering of positions with a similar color in space illustrates the fact that positions that are nearby in 1D tend to be nearby in 3D in a fractal globule. The simpler illustration at the right, showing the construction of the 3D Hilbert Curve, shows how a mathematical model of such a fractal globule can be constructed. In this iterative process, each line segment is folded into a seven-segment pattern with the same start- and end-point as the original segment. The resulting contour is precisely defined, fitting into the same initial volume vis-a-vis an increasingly tortuous trajectory, which is why such curves are known as “space-filling.” The Hilbert Curve was first constructed by the mathematician David Hilbert in 1891 (Hilbert, 1891). Inspired by the Hilbert curve, the fractal globule model for polymers such as the genome was first proposed in 1988 (Grosberg, Nechaev, & Shakhnovich, 1988). Empirical evidence in support of the model is provided in Lieberman-Aiden & Van Berkum et al., 2009.
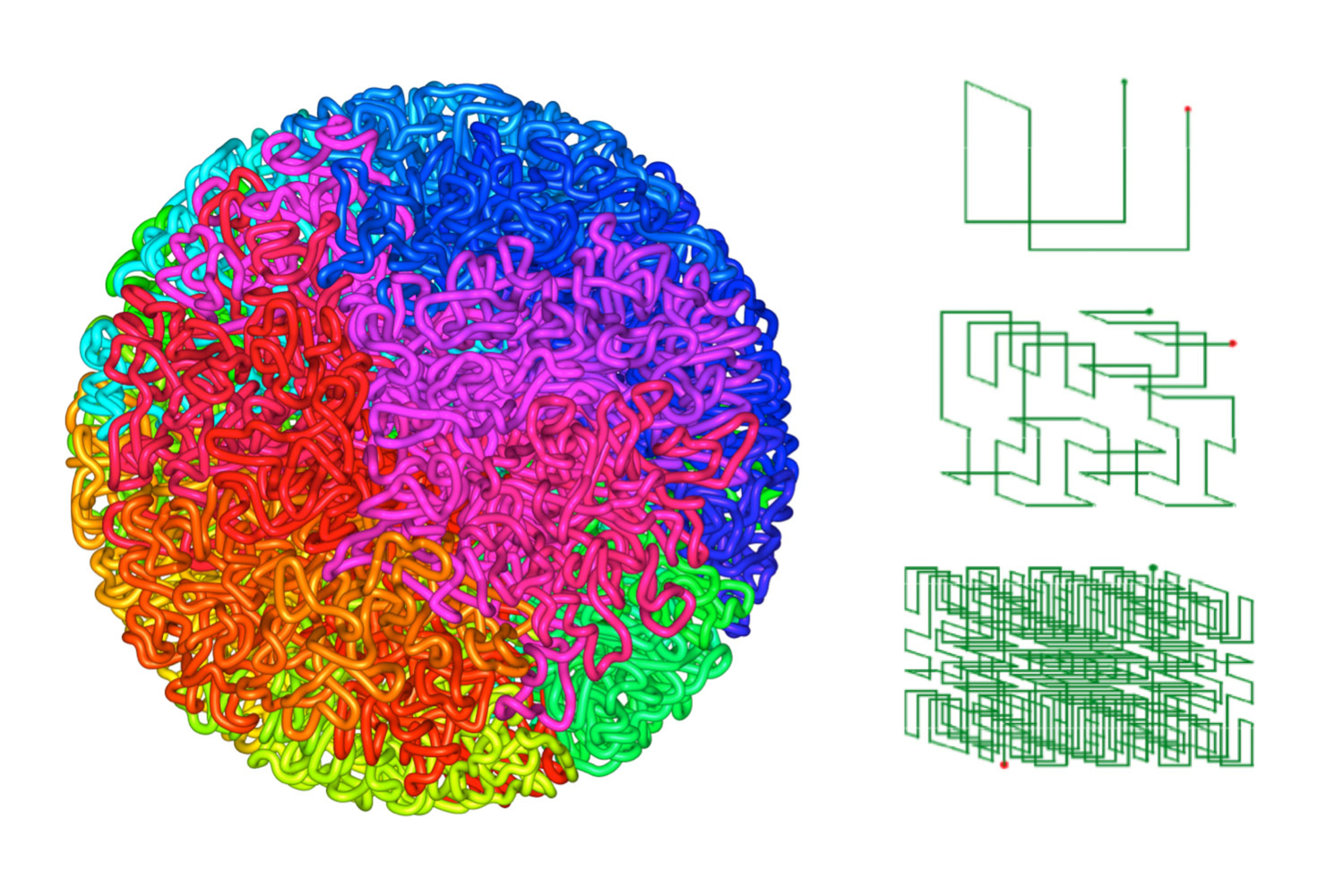
Left side: A ball made of dense, tightly folded and curving lines. Large sections of the ball are brightly colored. Right Side: Three line drawings, arranged in a vertical row, show lines that fold at right angles.
Left: Huntley, M. and Aiden, E.L. 2015. Image is based on work published in Sanborn & Rao et al., 2015b. Right: Aiden, E.L. 2009. Image first published in Lieberman-Aiden & Van Berkum et al., 2009. / - (2009)
Figure 2. The 2D Peano curve is constructed using an iterative procedure. In this procedure, each line segment is folded into eight segments, which preserve the start- and end-point while sweeping out an adjacent 2D area. In the mathematical limit - after the procedure is repeated infinitely many times - the curve touches every point inside the square. This raises the question of whether the curve, first constructed by Giuseppe Peano in 1890 (Peano, 1890), is one- or two-dimensional, or whether this notion of dimensionality is even meaningful. The original report introducing space-filling curves by Peano introduces the concept using mathematical language, but does not include a visualization of the curve. It is challenging to make this seminal concept intelligible and intuitive to the mind and senses. Motivated by Peano’s study, Hilbert’s 1891 contribution included a new, similar curve, complete with a diagram to assist the reader’s intuition.
Five square images in a horizontal row. In the leftmost one, a multicolored line folds at right angles. In each of the subsequent images, each segment of the line folds into equal segments, but the space occupied by the total line stays the same. As a result, the images to the right are increasingly dense and complex.
Aiden, E.L. / - (2009)

Erez: The objects that scientists study exist independently in the physical world, but the content of scientific theories is abstract. In order to reason about these theories and explore their implications, scientists must develop ways of representing theory. Thus, the problem of representation in science is not merely a problem of illustration, but an essential component of scientific reasoning.

Our laboratory's work on the three-dimensional structure of the human genome illustrates the challenges inherent in representing scientific concepts. Stretched end-to-end, the DNA molecules in a human cell would span two meters, but they are compressed into a space only a few microns wide. How can the human mind possibly conceive of such an extraordinary folding process?

Today, many disciplines are working together to answer this question. Mathematicians use idealized curves; physicists attempt to construct computer models; microscopists try to illuminate stretches of the genome. Gupi, too, used her particular toolset—the toolset of an artist—to work towards an answer. By directly engaging the senses, her media and her way-of-thinking opened up new avenues for understanding. Because her work is abstract—the intent is not to recapitulate a visible object—its intrinsic goal is to reveal things the eyes cannot ordinarily see. 

Exploration

Gupi: While Erez spoke about the problem of genome folding in scientific and mathematical terms, I saw the genome in visual, artistic terms—as a line with area and volume that takes on a form and a shape of its own. We spent time playing with electrical wire, trying to fold it into iterations of the Hilbert and Peano curves (Fig. 3). I used rectilinear and curvilinear interpretations based on the Hilbert and Peano curves to make drawings and prints (Fig. 4). While Erez concentrated on folding the wire, and referred to it as a puzzle, I was interested in unfolding the wire as it could take countless forms. I set out to explore the concept of "unfolding": as life unfolds, as the genome continuously moves and unfolds to interact and give instruction to the machinery of the cell, and as scientific discovery unfolds at the Broad Institute.

Figure 3. Forms created in attempts to iterate the Hilbert and Peano curves with electrical wire. While the image on the left shows a collection of wires folded in a physical attempt to understand different types of fractal curves, the two images on the right show the more successful attempts to recreate the folded Peano and Hilbert curves in a physical form.
Three images in a horizontal row show red and black electrical wire models on a white table. They are bent and folded in upon themselves, resulting in complex forms.
Ranganathan, G. / - (2009)
Figure 4. Ranganathan making prints based on the Hilbert and Peano curves in the Golub Lab at the Broad Institute of MIT and Harvard for the Unfolding project (2009-2011). The sets of prints to the left and right both focus on “unfolding.” The prints on the left use a rectilinear form of representation; a continuous S-shaped curve (similar to the 2-D Peano curve) runs through and connects all these prints. The prints on the right reflect experiments with electrical wire using both rectilinear and curvilinear forms of representation.
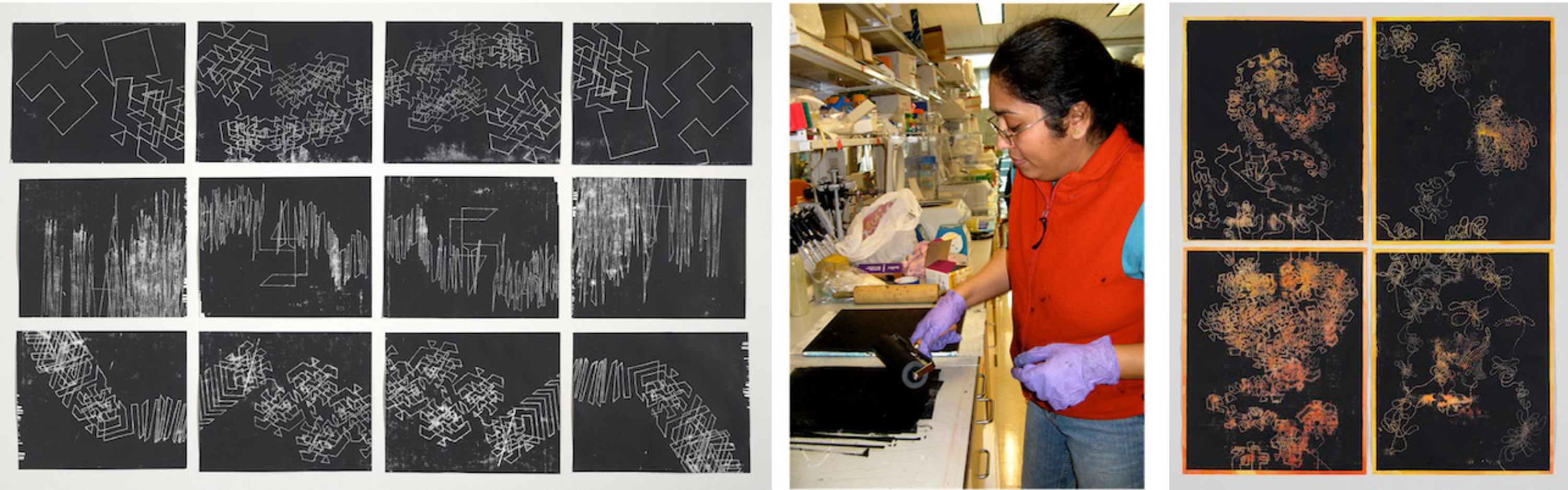
Three images in a horizontal row. The first is divided into an even grid of black squares, each one with a different complex, rectilinear or jagged pattern of whitish lines. These are woodblock prints. In the second image, the artist rolls ink for woodblock printing in the lab. She stands under long, cluttered shelves, using a small paint-roller on large black squares on a counter. She has black hair in a ponytail and wears a red quilted vest and jeans. The third image is divided into an even 2x2 grid of black squares—also woodblocks prints—each with complex, rectilinear and curving orange lines.
Howcroft, W / - (2010)

For almost a year, Erez and I made no break-through with the collaborative process. We were worried that I was creating drawings, prints, and artworks that illustrate but do not advance the project. Erez especially wondered why I kept wrestling with the electrical wires as I tried to assemble and create a physical model of the Peano and Hilbert curves. I felt that there was something important about the process of repeatedly folding and unfolding the electrical wires that I was missing. 

Summer 2010, I had my Aha! moment by myself while on a trip to the Ekambareswarar (Shiva) Temple in Kanchipuram, India, built in 600 CE. I was struck by two elements of the temple: the hundreds of pillars which appear identical at a distance but which reveal detailed variation up close, and the Shiva linga sculptures which are glimpsed between the pillars (Fig. 5). Like the pillars, each Peano curve is unique, but the distinctive variations are subtle and not immediately apparent. As for the sculptures, the Shiva linga is an abstract representation of God, the form of the formless. However, there also exist sculptures of the Shiva in human form performing the cosmic dance, and it is understood that both types of Shiva sculptures approach the same truth. Perhaps the mathematical models of the genome, floating in a software program, are like the Shiva linga, but there is another reality in which the genomic curve is a corporeal thing in motion, stretching and compressing. The whole line is intelligent; it has memory, and it can fold and unfold itself. Sculptures of the dancing Shiva each capture a different point in time in a dance and, even more, the sculptures are different proportions and sizes. Could I capture moments of the genome’s dance as the dancing Shiva sculptures do, pursuing the dynamic and physical form of something abstract? 

Figure 5. The 1000-pillar hall in the 600 CE Ekambareswarar (Shiva) Temple, Kanchipuram, India. Shiva linga sculptures are visible between pillars.
Two images side by side show a corridor lined on both sides with intricately carved pillars. Everything—floor, walls, pillars—is made of gray stone. In one image, a woman in a purple and gold sari sits at the foot of one of the pillars.
Ranganathan, G / - (2010)

I focused on the continuous movement of the genomic line as it folds and unfolds at the microscopic level. I could see that there can be infinite possibilities to folding the electrical wire, or the genomic section, while still complying with the requirements of the idealized, tangle-free Peano curve. Portraying the dynamic movement of a section of the genomic line as a sequence of poses or still images might communicate this complexity. Back in my studio, I quickly manipulated the wire held in my left hand, drawing on Post-Its the resulting silhouettes and forms in seven segments. Each fit uniquely into the two-dimensional square space, just as the genome, regardless of its number of segments, fits into the same miniscule space. The resulting 120 Post-Its is like a collection of animation movie stills; it takes some visual cues from The Simpsons, which I thought would resonate with American viewers (Fig. 6 and Fig. 7).

Figure 6. An early draft of Unfolding: 120 Post-Its (2010).
One hundred twenty Post-Its are stuck to a white surface in an even 8x15 grid. In each one, a thick black line folds at different angles, varying in shape and complexity from Post-It to Post-It.
Ranganathan, G / - (2010)
Figure 7. Site-specific installation of Unfolding: 120 of Infinite Possibilities. (2011)
One hundred twenty square wooden panels in a 12 X 10 grid on a black wall. In each one, a thick black line folds at different angles, varying in shape and complexity from panel to panel.
Hetherington, L B / - (2011)
Figure 8. Unfolding: Synthesis I, IV & V (2011). In these paintings, the lines from the 120 Post-Its are layered to explore the complexity of representing the dynamic 3-dimensional structure of the folded genome in 2 dimensions.
Three square images in a horizontal row. In each image, a black or silver line is folded upon itself to create a dense and complex form. From left to right, the forms are increasingly dense and complex. The background of each image is different color.
Howcroft, W / - (2018)

I began to wonder what we would see if we used a long armature wire, or multiple armature wires, with multiple 7-length segments, to create a physical genome model that could be compressed and stretched. I made a large sculptural model from eighteen coils of 20-foot armature wire, focusing on a dynamic structure with many possibilities, to see how it would inform the project. Over the next few months, I created paintings based on the 120 Post-Its (Fig. 8) and these wire structures, and folded the coils to create a suspended sculpture for Erez and the other researchers to interact with and explore (Fig. 9). 

Figure 9. Site-specific installation of Unfolding: 18 Coils. Wire coils are suspended under the Broad lobby stairway (2011-2012). Passersby were invited to manipulate the wire coils as they wished, and could see glimpses of the genomic sculpture through the treads as they climbed the stairs.
A wire sculpture made of tightly folded coils of armature wire hangs beneath a glass and metal stairway.
Hetherington, L B / - (2011)

The physical in conversation with the abstract

Gupi: 120 Post-Its opened up an entire world of possibilities for seeing, visual thinking, reasoning, linking, and understanding how the genome folds and unfolds—processes that Erez, the Aiden Lab, other researchers, and I had not considered before. I started seeing my work through new eyes, asking new questions and exploring possibilities beyond What is this? and Where is this going? to What if? and Maybe... As we both shifted the grids and perspectives that come with our separate disciplines, I created a series of mixed-media drawings, Sampling - Beyond Observation: Filling the Gaps (Fig. 10), to suggest some of the many possibilities for the invisible dynamic structure as the genome folds and unfolds in each cell of our body throughout our lives. Erez borrowed the digital images of these mixed-media drawings to continue his work on the problem of genome folding with the Aiden Lab. 

Figure 10. Unfolding: Sampling - Beyond Observation: Filling the Gaps (01-10) (2011).
Ten artworks. Most have one or more dark, round shapes loosely connected by densely curving or folding lines in front of a brightly colored background.
Ranganathan, G. (2011)

Erez: Gupi's massive, extraordinary wire sculptures, and more so the experience of watching her explore the problem of genome folding using the medium of electrical wire, was eye-opening for me. In these explorations, the world of invisible theories crashes into the world of our sensory experience. It made it possible to develop and deploy new sorts of sensory intuition, both visual and tactile, to a problem where our intuitions had typically come up short.

These physical explorations with Gupi’s artwork went hand-in-hand with our mathematical and physical explorations of our genome folding theories. A curve that might be first traced out in 3D space could, for example, be translated into an idealized mathematical trajectory, and from there, it might be examined using a new tool set. Conversely, a mathematical effort to examine the consequences of knotting in the genome could directly benefit from realizing the mathematical knot as a physical object which one could push, pull, stretch, and deform (Fig. 10).

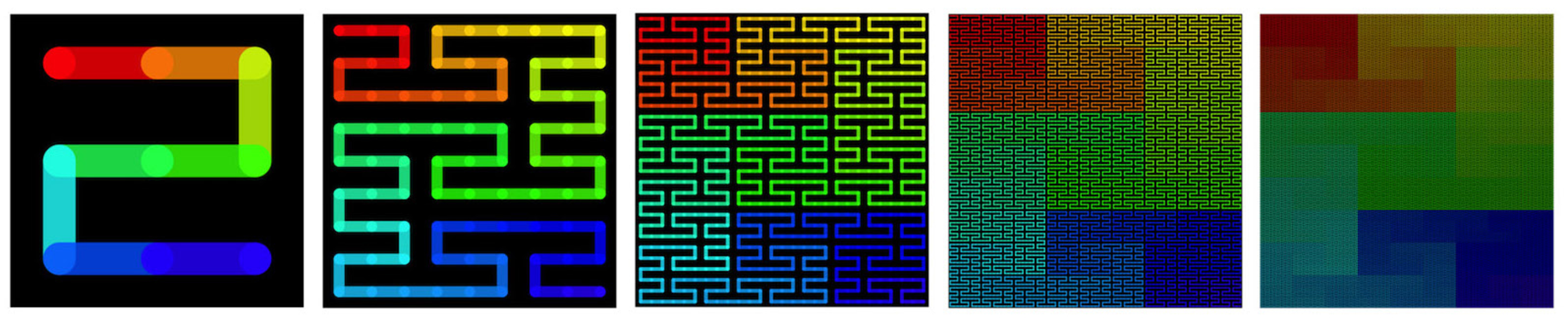
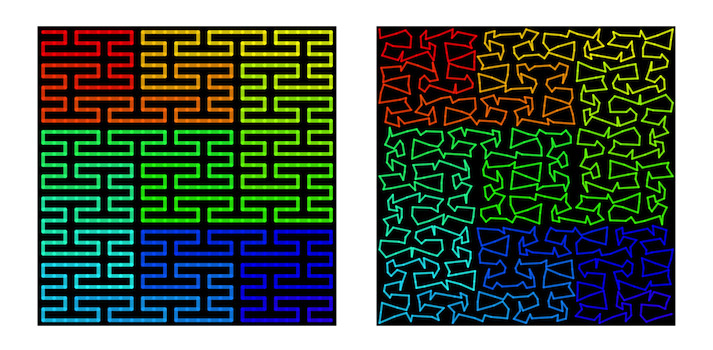
The creative process and Gupi’s serial visual experimentations led me and my team to explore mathematical variants on the curves we use to help model the genome, variants that we may not have otherwise emphasized. For instance, Figure 11A is a traditional representation of the Peano curve. Now, a careful observer will note that the curve is made of line segments with a fixed size. This sort of construction is mathematically natural, and easy to implement on a computer, but it is hard to do with electrical wire! Real materials—like electrical wire, but also like DNA—resist this sort of perfect homogeneity. Our work immediately raised the question: could we construct curves that reflect the heterogeneity of real objects, while retaining the dimension-defying mathematical equivalence to Peano’s curve? And could not such a curve be a superior model of the folded genome as compared to what we had used before? Figure 11B is (part of!) a mathematical response in the affirmative.

Figure 11. 11A (left side) is a traditional representation of the Peano curve, in which the curve is plotted at fixed intervals. 11B (right side) is an alternative representation, in which the curve is plotted at random intervals. This small change makes it more closely resemble a physical trajectory, although it is a visual manifestation of the very same mathematical object as in 11A.
Two square images side by side. The first is a multi-colored line that folds at regularly repeating 90-degree angles to form a dense pattern. The second is similarly dense and multi-colored, but here the line folds in regularly repeating sharp zig-zags.
Aiden, E. L. Construction details for the panel at right are given in Lieberman, 2010. / - (2011)

Art as manifestation of the possible

Erez: There is much about the folding of genomes that remains mysterious, about which we can only speculate. For instance, several years ago, our lab encountered what we called “superloops” in the genome which arise when stretches of DNA that are extraordinarily far apart along the contour of the chromosome encounter one another in 3D space (Darrow & Huntley et al., 2016). This is a phenomenon which we did not predict, which was hard to explain given our best theories, and whose underlying mechanism remains unknown even today. I suspect that Gupi's mixed-media drawings (Fig. 10) have long engaged with this interface, and we use them routinely as a way of visually evoking this unsettled state of affairs at the frontiers of our discipline (Darrow & Huntley et al., 2016; Rao et al, 2017; Rao & Huntley et al., 2014; Sanborn & Rao et al., 2015b). Here, the discrete, geometrical definiteness of the wire recedes somewhat, making space for something more indefinite: what happens when our simplistic models encounter the vast, unimaginable reality that lies just beyond our theory's grasp.

One of the most suggestive elements of the mixed media drawings is the way in which they provide a representation that integrates diverse modes of thought. In each image, one can observe coherent structures bubble up, as if from a structural welter—here, an idealized mathematical curve; there, the same curve but stretched, compressed, bent, warped—the ideal object as it manifests with real materials. Unlike a scientific diagram, the images are not didactic; they present possibilities without enforcing a single, rigid interpretation. In this respect they are more true to our understanding than any diagram that is presently possible.

Gupi: Focusing on the idea and concept of "unfolding" in my artworks enabled us to reframe our questions. I finally understood that what we had been doing was slowly and continuously shifting from the abstract ideal world of the Hilbert and Peano curves - to the representational ideal world of our initial electrical wire models, drawings, and prints - to the representational real world of the electric wires - to the abstract representations of the real world with 120 Post-Its - to the representational real world of the eighteen coils sculptural installation - and, finally, to the imagined and suggested world of the combined abstract and real mixed-media drawings. Our collaborative creative process had enabled me to “see” that we are following the invisible; the suggested worlds made visible in my art are in a back-and-forth conversation with the advances in Erez’s lab, revealing directions for our shared journey we hadn’t envisioned (Fig. 12). 

Figure 12. Installation of Unfolding: Sampling: Beyond Observation: Following the Invisible (2011).
A large, rectangular, mixed-media artwork displayed on a bright orange background. The artwork has a complicated profusion of tightly curving lines and overlapping shapes.
Hetherington, L.B. (2011)

Supplemental material

Unfolding 2011. 120 Post-its also led to the creation of an animation video of a compressed genome as a dot opening up to form a genomic line giving instructions and folding again to form a dot. In 2011, I worked with Lars Erik-Siren (Broad Institute Communications team) to create a looping animation video. I combined soundtracks of wind and percussion instruments that follow the mapped migratory routes of the Genographic Project (IBM, 2011), and used the lines drawn with Aiden on the problem of genome folding to narrate an abstract story of human DNA, migration, evolution, research and understanding. This video is available through the Broad Institute: https://www.youtube.com/watch?v=Aj_trjZ7jKE
(2011) - https://www.youtube.com/watch?v=Aj_trjZ7jKE

Bibliography and further reading

x

Acknowledgements

Broad Institute of MIT and Harvard, Cambridge, MA



Completed

 Between September 2009 and December 2011

Website:

 Project Site

Sites and Institutes

 Broad Artist-in-Residence Program, Broad Institute of MIT and Harvard
Aiden Lab, The Center for Genome Architecture, Baylor College of Medicine, Rice University

Keywords

 Art + Science Collaboration Art As Research

Disciplines

 Art Genomic Research Applied Math Biology

Views

 1467 views *